1

The cost of a notebook is twice the cost of a pen. Write a linear equation in two variables to represent this statement.
(Take the cost of a notebook to be x and that of a pen to be y).

Answer

Let the cost of a notebook = ₹ x
The cost of a pen = ₹ y
According to the condition,
we have [Cost of a notebook] = 2 x [Cost of a pen]
i.e. [x] = 2 x [y]
or
x = 2y
or
x – 2y = 0
Thus, the required linear equation is x – 2y = 0.

Exercise 4.1 Page Number 68

2(i)

Express the following linear equations in the form ax + by + c = 0 and indicate the values of a, b and c in each case:
2x + 3y =9.3

Answer

We have 2x + 3y = 9.3
∴ (2)x + (3)y + (–9.3) = 0
Comparing it with ax + bx + c = 0,
we have a = 2, b = 3 and c = –9.3.

Exercise 4.1 Page Number 68

2(ii)

Express the following linear equations in the form ax + by + c = 0 and indicate the values of a, b and c in each case:
x – (y/5) – 10 = 0

Answer

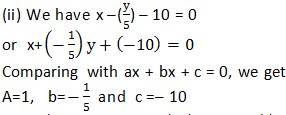
Note: Above equation can also be compared by:
Multiplying throughout by 5,

or 5(x) + (–1)y + (–50) = 0
Comparing with ax + by + c = 0,
we get a = 5, b = –1 and c = –50.

Exercise 4.1 Page Number 68

2(iii)

Express the following linear equations in the form ax + by + c = 0 and indicate the values of a, b and c in each case:
–2x + 3y = 6

Answer

We have –2x + 3y = 6
⇒ –2x + 3y – 6 = 0
⇒ (–2)x + (3)y + (–6) = 0
Comparing with ax + bx + c = 0,
we get a = –2, b = 3 and c = –6.

Exercise 4.1 Page Number 68

2(iv)

Express the following linear equations in the form ax + by + c = 0 and indicate the values of a, b and c in each case:
x = 3y

Answer

We have x = 3y
⇒ x – 3y = 0
⇒ (1)x + (–3)y + 0 = 0
Comparing with ax + bx + c = 0,
we get a = 1, b = –3 and c = 0.

Exercise 4.1 Page Number 68

2(v)

Express the following linear equations in the form ax + by + c = 0 and indicate the values of a, b and c in each case:
2x = –5y

Answer

We have 2x = –5y
⇒ 2x + 5y = 0
⇒ (2)x + (5)y + 0 = 0
Comparing with ax + by + c = 0,
we get a = 2, b = 5 and c = 0.

Exercise 4.1 Page Number 68

2(vi)

Express the following linear equations in the form ax + by + c = 0 and indicate the values of a, b and c in each case:
3x + 2 = 0

Answer

We have 3x + 2 = 0
⇒ 3x + 2 + 0y = 0
⇒ (3)x + (0)y + (2) = 0
Comparing with ax + by + c = 0,
we get a = 3, b = 0 and c = 2.

Exercise 4.1 Page Number 68

2(vii)

Express the following linear equations in the form ax + by + c = 0 and indicate the values of a, b and c in each case:
y – 2 = 0

Answer

We have y – 2 = 0
⇒ (0)x + (1)y + (–2) = 0
Comparing with ax + by + c = 0,
we have a = 0, b = 1 and c = –2.

Exercise 4.1 Page Number 68

2(viii)

Express the following linear equations in the form ax + by + c = 0 and indicate the values of a, b and c in each case:
5 = 2x

Answer

We have 5 = 2x
⇒ 5 – 2x = 0
⇒ –2x + 0y + 5 = 0
⇒ (–2)x + (0)y + (5) = 0
Comparing with ax + by + c = 0,
we get a = –2, b = 0 and c = 5.

Exercise 4.1 Page Number 68

1

Which one of the following options is true, and why ? y = 3x + 5 has
(i) a unique solution,
(ii) only two solutions,
(iii) infinitely many solutions

Answer

Option (iii) is true because a linear equation has an infinitely many solutions.

Exercise 4.2 Page Number 70

2

Write four solutions for each of the following equations:
(i) 2x + y = 7
(ii) πx + y = 9
(iii) x = 4y

Answer

(i) 2x + y = 7
When x = 0, 2(0) + y = 7
⇒ 0 + y = 7
⇒ y= 7
∴ Solution is (0, 7).
When x = 1, 2(1) + y = 7
⇒ y = 7 – 2
⇒ y= 5
∴ Solution is (1, 5).
When x = 2, 2(2) + y = 7
⇒ y = 7 – 4
⇒ y = 3
∴ Solution is (2, 3).
When x = 3 2(3) + y = 7 ⇒ y = 7 – 6
⇒ y = 1
∴ Solution is (3, 1).

(ii) πx + y = 9
When x = 0 π(0) + y = 9
⇒ y = 9 – 0
⇒ y= 9
∴ Solution is (0, 9).
When x = 1, π(1) + y = 9
⇒ y = 9 – π
∴ Solution is {1, (9 – π)}.
When x = 2 π(2) + y = 9
⇒ y = 9 – 2π
∴ Solution is {2, (9 – 2π)}.
When x = –1, p(–1) + y = 9
⇒ –π + y = 9
⇒ y = 9 + π
∴ Solution is {–1, (9 + π)}.

(iii) x = 4y When x = 0, 4y = 0
⇒ y = 0
∴ Solution is (0, 0).
When x = 1, 4y = 1
⇒ y = (1/4)
∴ Solution is (1, (1/4))
When x = 4, 4y = 4
⇒ y= (4/4) = 1
∴ Solution is (4, 1).
When x = –4, 4y = –4
⇒ y = (-4/4) = –1
∴ Solution is (–4, –1).

Exercise 4.2 Page Number 70

3

Check which of the following are solutions of the equation x - 2y = 4 and which are not:
(i) (0, 2)
(ii) (2, 0)
(iii) (4, 0)
(iv) (√2, 4√2)
(v) (1, 1)

Answer

(i) (0, 2) means x = 0 and y = 2
Putting x = 0 and y = 2 in x – 2y = 4,
we have L.H.S. = 0 – 2(2) = –4
But R.H.S. = 4
∴ L.H.S. ≠ R.H.S.
∴ x = 0, y = 0 is not a solution.

(ii) (2, 0) means x = 2 and y = 0
∴ Putting x = 2 and y = 0 in x – 2y = 4,
we get L.H.S. = 2 – 2(0) = 2 – 0 = 2
But R.H.S. = 4
∴ L.H.S. ≠ R.H.S.
∴ (2, 0) is not a solution.

(iii) (4, 0) means x = 4 and y = 0
Putting x = 4 and y = 0 in x – 2y = 4,
we get L.H.S. = 4 – 2(0) = 4 – 0 = 4
But R.H.S. = 4
∴ L.H.S. = R.H.S.
∴ (4, 0) is a solution.

(iv) (2 , 4√2) means x = 2 and y = 4√2
Putting x = 2 and y = 4√2 in x – 2y = 4,
we get L.H.S. =√2 – 2 ( 4√2) = √2 – 8√2
= √2(1 – 8) = –7√2
But R.H.S. = 4 ∴ L.H.S. ≠ R.H.S.
∴ (√2, 4√2) is not a solution.

(v) (1, 1) means x = 1 and y = 1
Putting x = 1 and y = 1 in x – 2y = 4,
we get L.H.S. = 1 – 2(1) = 1 – 2 = –1
But R.H.S. = 4
⇒ L.H.S. ≠ R.H.S
∴ (1, 1) is not a solution.

Exercise 4.2 Page Number 70

4

Find the value of k, if x = 2, y = 1 is a solution of the equation 2x + 3y = k.

Answer

We have 2x + 3y = k
Putting x = 2 and y = 1 in 2x + 3y = k,
we get 2(2) + 3(1) = k
⇒ 4 + 3 = k
⇒ 7 = k
Thus, the required value of k = 7.

Exercise 4.2 Page Number 70

1

Draw the graph of each of the following linear equations in two variables:
(i) x + y = 4
(ii) x - y = 2
(iii) y = 3x
(iv) 3 = 2x + y

Answer

(i)x + y= 4
⇒ y = 4 – x
If we have x = 0,
then y = 4 – 0 = 4 x = 1,
then y = 4 – 1 = 3 x = 2,
then y = 4 – 2 = 2
∴ We get the following table:

x	0	1	2
y	4	3	2

Plot the ordered pairs (0, 4), (1, 3) and (2, 2) on the graph paper. Joining these points, we get a line AB as shown below.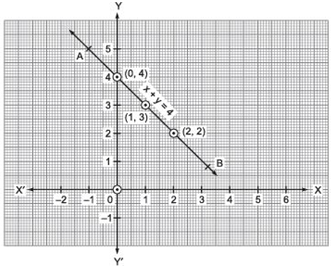

Thus, the line AB is the required graph of x + y = 4.

(ii) x – y = 2
⇒ y = x – 2
If we have x = 0,
then y = 0 – 2 = –2 x = 1,
then y = 1 – 2 = – 1 x = 2,
then y = 2 – 2 = 0
∴ We have the following table:

x	0	1	2
y	-2	-1	0

Plot the ordered pairs (0, –2), (1, –1) and (2, 0) on the graph paper. Joining these points, we get a straight line PQ as shown below: Thus, the line PQ is required graph of x – y = 2.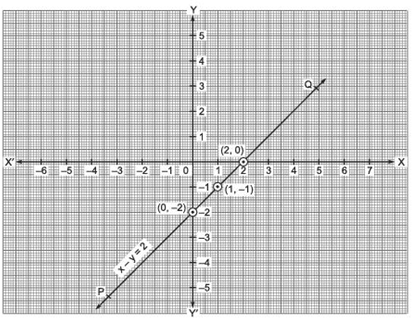

(iii) y = 3x
If x = 0, then y = 3(0)
⇒ y = 0 x = 1, then y = 3(1)
⇒ y = 3 x = –1, then y = 3(–1)
⇒ y = –3
∴ We get the following table:

x	0	1	-1
y	0	3	-3

Plot the ordered pairs (0, 0), (1, 3) and (–1, –3) on the graph paper. Joining these points, we get the straight line LM.
Thus, LM is the required graph of y = 3x.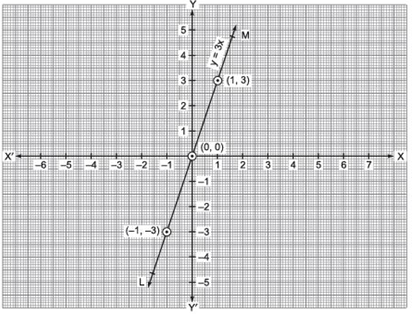

Note: The graph of the equation of the form y = kx is a straight line which always passes through the origin.

( iv) 3 = 2x + y
⇒ y = 3 – 2x
∴ If x = 0, then y = 3 – 2(0)
⇒ y = 3 If x = 1, then y = 3 – 2(1)
⇒ y = 1 If x = 2, then y = 3 – (2)
⇒ y = –1

x	0	1	2
y	3	1	-1

Plot the ordered pairs (0, 3), (1, 1) and (2, –1) on the graph paper. Joining these points, we get a line CD.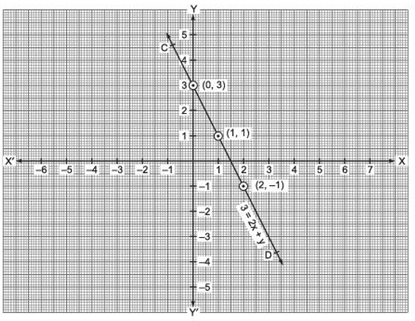

Thus, the line CD is the required graph of 3 = 2x + y

Exercise 4.3 Page Number 74

2

Give the equations of two lines passing through (2, 14). How many more such lines are there, and why?

Answer

(2, 14) means x = 2 and y = 14
Following equations can have (2, 14) as the solution, i.e. they can pass through the point (2, 14).
(i) x + y = 16
(ii) 7x – y = 0
There can be an unlimited number of lines which can pass through the point (2, 14) because an unlimited number of lines can pass through a point.

Exercise 4.3 Page Number 74

3

If the point (3, 4) lies on the graph of the equation 3y = ax + 7, find the value of a.

Answer

The equation of the given line is 3y = ax + 7
∵ (3, 4) lies on the given line.
∴ It must satisfy the equation 3y = ax + 7
We have (3, 4)
⇒ x = 3 and y = 4
L.H.S. = 3y
= 3 x 4
= 12
R.H.S. = ax + 7
= a x 3 + 7
= 3a + 7
∵ L.H.S. = R.H.S.
∴ 12 = 3a + 7
or
3a = 12 – 7 = 5
or
a = (5/3)
Thus, the required value of a is (5/3).

Exercise 4.3 Page Number 74

4

The taxi fare in a city is as follows:
For the first kilometre, the fare is ₹ 8 and for the subsequent distance it is ₹ 5 per km. Taking the distance covered as x km and total fare as ₹ y, write a linear equation for this information and draw its graph.

Answer

Here, total distance covered = x km
Total taxi fare = ₹ y
Fare for the 1st km = ₹ 8
Remaining distance = (x – 1) km
∴ Fare for (x – 1) km = ₹ 5 x (x – 1) km
Total taxi fare = ₹ 8 + ₹ 5(x – 1)
∴ According to the condition,
y = 8 + 5(x – 1)
⇒ y = 8 + 5x – 5
⇒ y = 5x + 3
which is the required linear equation representing the given information.
Graph: We have y = 5x + 3
∴ When x = 0, y = 5(0) + 3
⇒ y = 3
When x = –1, y = 5(–1) + 3
⇒ y = –2
When x = –2, y = 5(–2) + 3
⇒ y = –7
∴ We get the following table:

x	0	-1	-2
y	3	-2	-7

Now, plotting the ordered pairs (0, 3), (–1, –2) and (–2, –7) on a graph paper and joining them, we get a straight line PQ.
Thus, PQ is the required graph of the linear equation y = 5x + 3.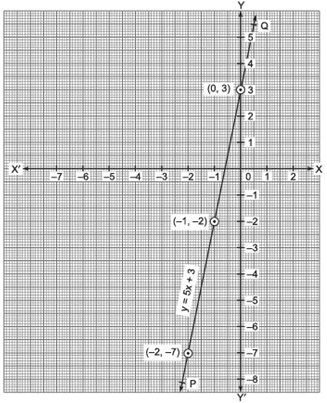

Exercise 4.3 Page Number 74

5

From the choices given below, choose the equation whose graphs are given in Fig. (i) and Fig.(ii).

For Fig. (i)

For Fig. (ii)

(i) y = x

(i) y = x + 2

(ii) x + y = 0

(ii) y = x – 2

(iii) y = 2x

(iii) y = –x + 2

(iv) 2 + 3y = 7x

(iv) x + 2y = 6

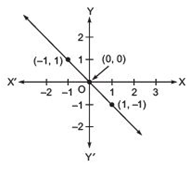
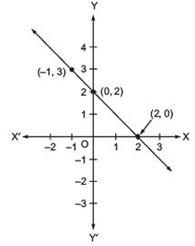
Answer

For Fig. (i), the correct linear equation is x + y = 0
[∵ (–1, 1) ⇒ –1 + 1 = 0 and (1, –1)
⇒ 1 + (–1) = 0]

For Fig. (ii), the correct linear equation is y = –x + 2
[∵ (–1, 3) ⇒ 3 = –(–1) + 2 ⇒ 3 = 3 and (0, 2)
⇒ 2 = –(0) + 2 ⇒ 2 = 2]

Exercise 4.3 Page Number 74

6

If the work done by a body on application of a constant force is directly proportional to the distance travelled by the body, express this in the form of an equation in two variables and draw the graph of the same by taking the constant force as 5 units. Also read from the graph the work done when the distance travelled by the body is
(i) 2 units (ii) 0 unit

Answer

Constant force is 5 units.
Let the distance travelled = x units and work done = y units.
Since, Work done = Force x Displacement
⇒ y= 5 × X
⇒ y= 5x
Drawing the graph
We have y = 5x
When x = 0, then y = 5(0) = 0
When x = 1, then y = 5(1) = 5
When x = 1.5, then y = 5(1.5) = 7.5
∴ We get the following table:

x	0	1	1.5
y	0	5	7.5

Plotting the ordered pairs (0, 0), (1, 5) and (1.5, 7.5) on the graph paper and joining the points, we get a straight line OB.

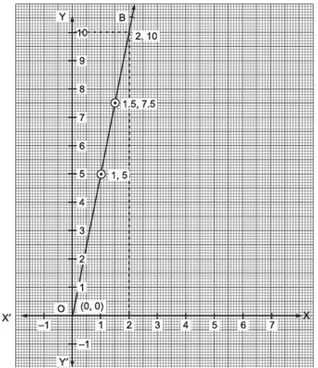
From the graph, we get

(i) Distance travelled = 2 units
∴ x = 2, then y = 10 units
⇒ Work done = 10 units.

(ii) Distance travelled = 0 units
∴ y = 5x
⇒ y = 5(0) = 0
∴ Work done = 0 unit.

Exercise 4.3 Page Number 75

7

Yamini and Fatima, two students of Class IX of a school, together contributed ₹ 100 towards the Prime Minister’s Relief Fund to help the earthquake victims. Write a linear equation which satisfies this data. (You may take their contributions as ₹ x and ₹ y.) Draw the graph of the same.

Answer

Let the contribution of Yamini = ₹ x and the contribution of Fatima = ₹ y
∴ We have x + y = 100
⇒ y = 100 – x
Now, when x = 0, y = 100 – 0 = 100
When x = 50, y = 100 – 50 = 50
When x = 100, y = 100 – 100 = 0
We get the following table:

x	0	50	100
y	100	50	0

For drawing the graph, plot the ordered pairs (0, 100), (50, 50) and (100, 0) on a graph paper.
Joining these points we get a line PQ.
Thus, PQ is the required graph of x + y = 100.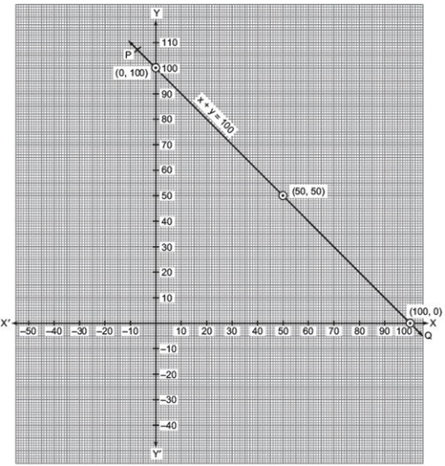

Exercise 4.3 Page Number 75

8

In countries like USA and Canada, temperature is measured in Fahrenheit, whereas in countries like India, it is measured in Celsius. Here is a linear equation that converts Fahrenheit to Celsius.
F= (9/5)C + 32
(i) Draw the graph of the linear equation above using Celsius for x-axis and Fahrenheit for y-axis.
(ii) If the temperature is 30ºC, what is the temperature in Fahrenheit?
(iii) If the temperature is 95ºF, what is the temperature in Celsius?
(iv) If the temperature is 0ºC, what is the temperature in Fahrenheit and if the temperature is 0ºF, what is the temperature in Celsius?
(v) Is there a temperature which is numerically the same in both Fahrenheit and Celsius? If yes, find it.

Answer

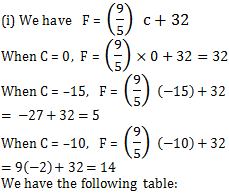
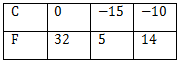
Plot the ordered pairs (0, 32), (–15, 5) and (–10, 14) on a graph paper. Joining these points we get a straight line AB.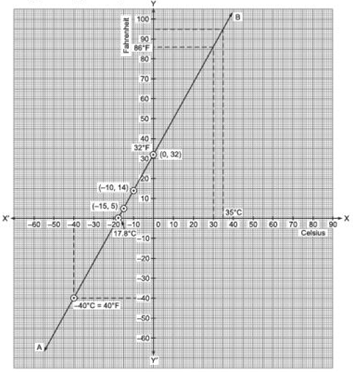

(ii) From the graph, we have
86ºF corresponds to 30ºC

(iii) From the graph, we have
95ºF = 35ºC

(iv) From the graph, we have
0ºC = 32ºF
and 0ºF = 17.8ºC

(v) Yes, from the graph, we have
40ºF = –40ºC

Exercise 4.3 Page Number 75

1

Give the geometric representations of y = 3 as an equation
(i) in one variable
(ii) in two variables

Answer

(i) y = 3 [An equation in one variable]
∵ y = 3 is an equation in one variable, i.e. y only.
∴ It has a unique solution y = 3 as shown on the number line shown here.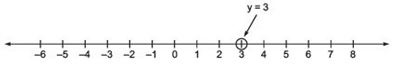

The unique solution is a point.

(ii) y = 3 [An equation in two variables]
We can write y = 3 as
0.x + y = 3
Now, when x = 1, y = 3
when x = 2, y = 3
when x = 3, y = 3
We get the following table: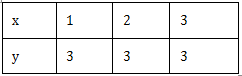

Plotting the ordered pairs (1, 3), (2, 3) and (3, 3) on a graph paper, we get a line AB as solution of 0 . x + y = 3, i.e. y = 3.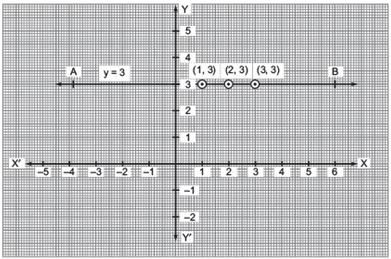

Exercise 4.4 Page Number 77

2

Give the geometric representations of 2x + 9 = 0 as an equation
(i) in one variable
(ii) in two variables

Answer

(i) 2x + 9 = 0 [An equation in one variable]
We have: 2x + 9 = 0
⇒ 2x = –9
⇒ x = (-9/2)
which is a linear equation in one variable ‘x’ only. Its solution is the point (-9/2) on the number line as shown below.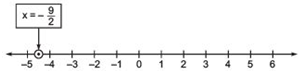

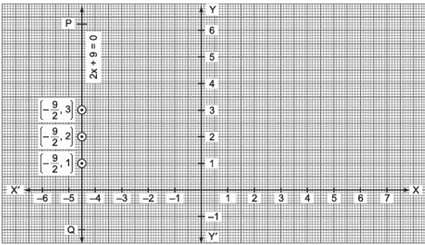
(ii) 2x + 9 = 0 [An equation in two variables]
We can write 2x + 9 = 0 as 2x + 0y + 9 = 0 or 2x = –9 + 0y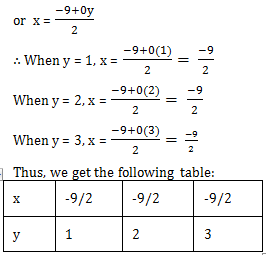

Now, plotting the ordered pairs on a graph paper and joining them, we get a line PQ as solution of 2x + 9 = 0.

Exercise 4.4 Page Number 77

Linear Equations in Two Variables